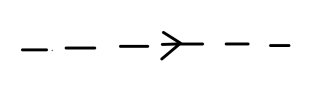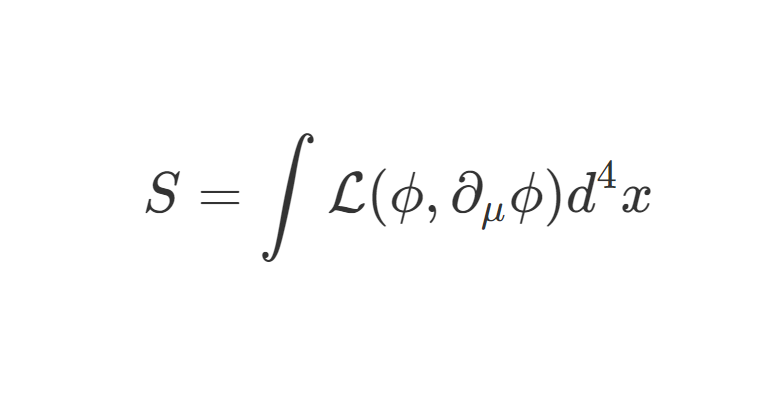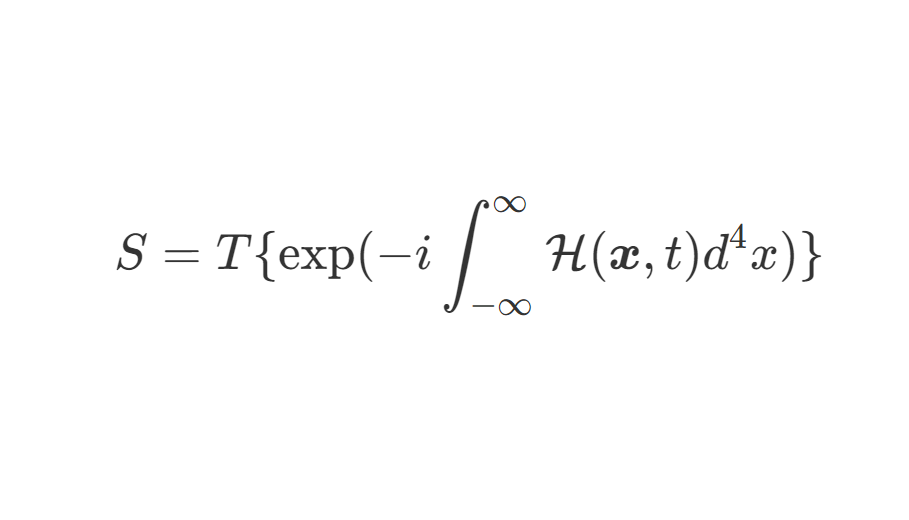费米子的费曼规则

首先为了应用 wick 定理，我们需要将时序符号进行推广，对于费米子场量 ψ(x)\psi(x) 来说：

T(ψ(x)ψˉ(y))≡{ψ(x)ψˉ(y)x0>y0−ψˉ(y)ψ(x)x0<y0(1)T(\psi(x)\bar{\psi}(y)) \equiv \left\{\begin{aligned} &\psi(x)\bar{\psi}(y)&\quad x^0>y^0\\ -&\bar{\psi}(y)\psi(x)&\quad x^0<y^0\\ \end{aligned}\right. \tag{1}

那么 Dirac 场的费米传播子可以表示为：

SF(x−y)=∫d4p(2π)4i(p+m)p2−m2+iϵe−ip⋅(x−y)=⟨0∣Tψ(x)ψˉ(y)∣0⟩(2)\begin{aligned} S_F(x-y) &= \int \frac{d^4p}{(2\pi)^4}\frac{i(\cancel{p}+m)}{p^2-m^2 + i\epsilon} e^{-ip\cdot(x-y)}\\ &= \langle 0|T\psi(x)\bar{\psi}(y)|0\rangle \end{aligned} \tag{2}

同样的，我们要推广正规序。注意，此时由于 Dirac 场阶梯算子的反对易关系，存在多种不同的正规序表示方式。例如：

N(apaqar†)=(−1)2ar†apaq=(−1)3ar†aqap(3)N(a_{\bm{p}}a_{\bm{q}}a_{\bm{r}}^\dagger) = (-1)^2a_{\bm{r}}^\dagger a_{\bm{p}}a_{\bm{q}} = (-1)^3 a_{\bm{r}}^\dagger a_{\bm{q}}a_{\bm{p}} \tag{3}

每一次相邻的阶梯算子的置换需要添加一个负号。

对于 Dirac 场来说，其中：

ψ+(x)=∫d3p(2π)312Ep∑sapsus(p)e−ip⋅xψ−(x)=∫d3p(2π)312Ep∑sbps†vs(p)eip⋅xψˉ+(x)=∫d3p(2π)312Ep∑sbpsvˉs(p)e−ip⋅xψˉ−(x)=∫d3p(2π)312Ep∑saps†uˉs(p)eip⋅x(4)\begin{aligned} \psi^+(x) &= \int \frac{d^3p}{(2\pi)^3} \frac{1}{\sqrt{2E_{\bm{p}}}}\sum_s a_{\bm{p}}^s u^s(\bm{p}) e^{-ip\cdot x}\\ \psi^-(x) &= \int \frac{d^3p}{(2\pi)^3} \frac{1}{\sqrt{2E_{\bm{p}}}}\sum_s b_{\bm{p}}^{s\dagger} v^s(\bm{p}) e^{ip\cdot x}\\ \bar{\psi}^+(x) &= \int \frac{d^3p}{(2\pi)^3} \frac{1}{\sqrt{2E_{\bm{p}}}}\sum_s b_{\bm{p}}^s \bar{v}^s(\bm{p}) e^{-ip\cdot x}\\ \bar{\psi}^-(x) &= \int \frac{d^3p}{(2\pi)^3} \frac{1}{\sqrt{2E_{\bm{p}}}}\sum_s a_{\bm{p}}^{s\dagger} \bar{u}^s(\bm{p}) e^{ip\cdot x}\\ \end{aligned}\tag{4}

对于 x0>y0x^0>y^0 的情况：

T(ψ(x)ψˉ(y))=x0>y0ψ+(x)ψˉ+(y)+ψ+(x)ψˉ−(y)+ψ−(x)ψˉ+(y)+ψ−(x)ψˉ−(y)(5)\begin{aligned} &T(\psi(x)\bar{\psi}(y))\overset{x^0>y^0}{=} & \psi^{+}(x)\bar{\psi}^{+}(y) + \psi^{+}(x)\bar{\psi}^{-}(y) + \psi^{-}(x)\bar{\psi}^{+}(y) + \psi^{-}(x)\bar{\psi}^{-}(y)\\ \end{aligned}\tag{5}

而：

N(ψ(x)ψˉ(y))=ψ+(x)ψˉ+(y)−ψˉ−(y)ψ+(x)+ψˉ+(y)ψ−(x)+ψ−(x)ψˉ−(y)(6)\begin{aligned} &N(\psi(x)\bar{\psi}(y))= & \psi^{+}(x)\bar{\psi}^{+}(y) - \bar{\psi}^{-}(y)\psi^{+}(x) + \bar{\psi}^{+}(y)\psi^{-}(x) + \psi^{-}(x)\bar{\psi}^{-}(y)\\ \end{aligned}\tag{6}

对比得到：

T(ψ(x)ψˉ(y))=x0>y0N(ψ(x)ψˉ(y))+{ψ+(x),ψˉ−(y)}T(\psi(x)\bar{\psi}(y))\overset{x^0>y^0}{=} N(\psi(x)\bar{\psi}(y)) + \{\psi^{+}(x),\bar{\psi}^{-}(y)\}

类似的，对于 x0<y0x^0<y^0 的情况，可以得到：

T(ψ(x)ψˉ(y))=x0<y0N(ψ(x)ψˉ(y))−{ψˉ+(y),ψ−(x)}T(\psi(x)\bar{\psi}(y))\overset{x^0<y^0}{=} N(\psi(x)\bar{\psi}(y)) -\{\bar{\psi}^+(y),\psi^-(x)\}

这样，我们定义费米子场间的缩并：

ψ∙(x)ψˉ∙(y)={{ψ+(x),ψˉ−(y)}x0>y0−{ψˉ+(y),ψ−(x)}x0<y0(7)\begin{aligned} \psi^\bullet(x)\bar{\psi}^\bullet(y) &= \left\{ \begin{aligned} &\{\psi^+(x),\bar{\psi}^-(y)\}&\quad x^0>y^0\\ -&\{\bar{\psi}^+(y),\psi^-(x)\}&\quad x^0<y^0\\ \end{aligned} \right.\\ \end{aligned}\tag{7}

其值正是 Dirac 场传播子，见 量子场论笔记（七）：Dirac 场的量子化。

ψ∙(x)ψˉ∙(y)=SF(x−y)(8)\psi^\bullet(x)\bar{\psi}^\bullet(y) = S_F(x-y) \tag{8}

那么有：

T(ψ(x)ψˉ(y))=N(ψ(x)ψˉ(y))+ψ∙(x)ψˉ∙(y)(9)T(\psi(x)\bar{\psi}(y)) = N(\psi(x)\bar{\psi}(y)) + \psi^\bullet(x)\bar{\psi}^\bullet(y) \tag{9}

对于 ψ,ψ\psi,\psi 或者 ψˉ,ψˉ\bar{\psi},\bar{\psi} 间的缩并，不难得到：

ψ∙(x)ψ∙(y)=ψˉ∙(x)ψˉ∙(y)=0(10)\psi^\bullet(x)\psi^\bullet(y) = \bar{\psi}^\bullet(x)\bar{\psi}^\bullet(y) = 0 \tag{10}

在进行缩并计算时，我们需要考虑符号，例如：

N(ψ1∙ψ2ψˉ3∙ψˉ4)=−ψ1∙ψ3∙N(ψ2ψˉ4)=−SF(x1−x3)N(ψ2ψˉ4)N(\psi_1^\bullet\psi_2\bar{\psi}_3^\bullet\bar{\psi}_4) = -\psi_1^\bullet\psi_3^\bullet N(\psi_2\bar{\psi}_4) = -S_F(x_1-x_3)N(\psi_2\bar{\psi}_4)

对于费米子来说，我们同样也有 wick 定理：

T[ψ1ψˉ2ψ3⋯ ]=N[ψ1ψˉ2ψ3⋯+ all possible contractions](11)T[\psi_1\bar{\psi}_2\psi_3\cdots] = N[\psi_1\bar{\psi}_2\psi_3\cdots + \ all\ possible\ contractions] \tag{11}

Yukawa 理论

我们现在分析 Yukawa 理论 的散射过程，Yukawa 理论是一个 量子电动力学 Quantum Electrodynamics(QED) 的简化模型，我们这里的讨论将为之后的 QED 做铺垫。Yukawa 理论的哈密顿量写为：

H=HDirac+HKlein−Gordon+∫d3xgψˉψϕ(12)H = H_{Dirac} + H_{Klein-Gordon} + \int d^3x g\bar{\psi}\psi\phi\tag{12}

其中 HDirac,HKlein−GordonH_{Dirac},H_{Klein-Gordon} 分别为自由 Dirac 场与 Klein-Gordon 场的哈密顿量，而第三项将这两个场耦合起来，表示相互作用，我们将这一项看成微扰。

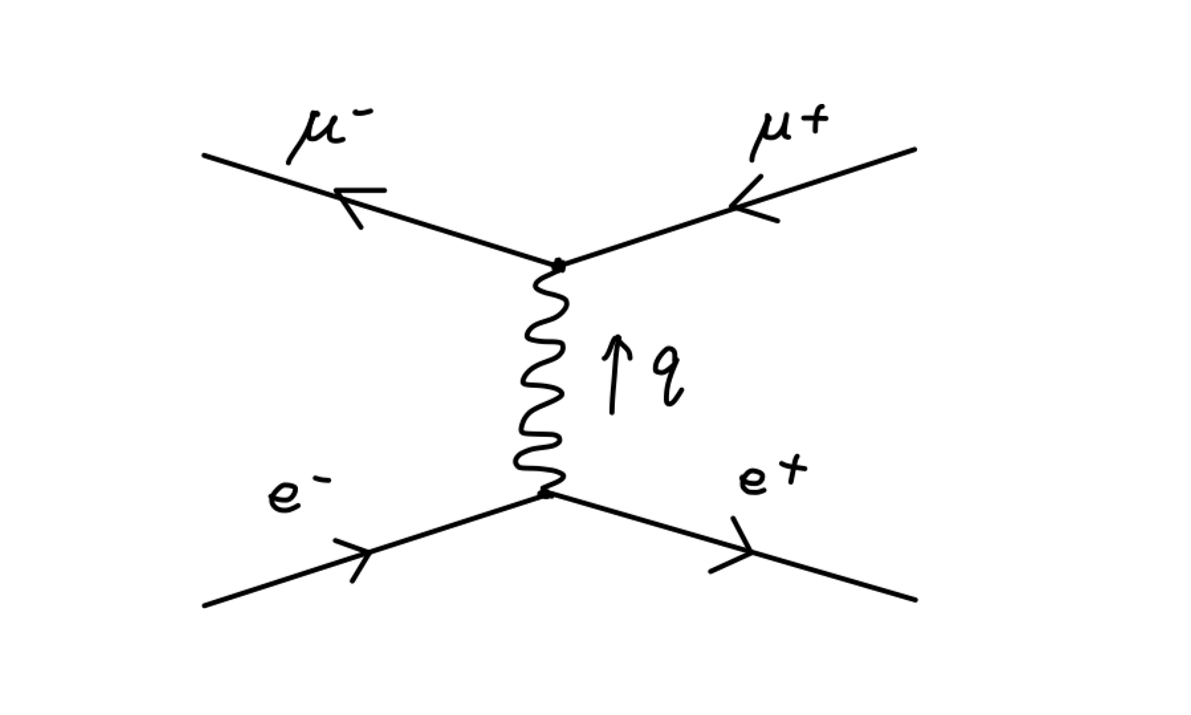
我们现在考虑如下的两个费米子的散射过程：

fermion(p)+fermion(k)→fermion(p′)+fermion(k′)fermion(p) + fermion(k) \rightarrow fermion(p') + fermion(k')

对散射矩阵的主要贡献项是二阶项：

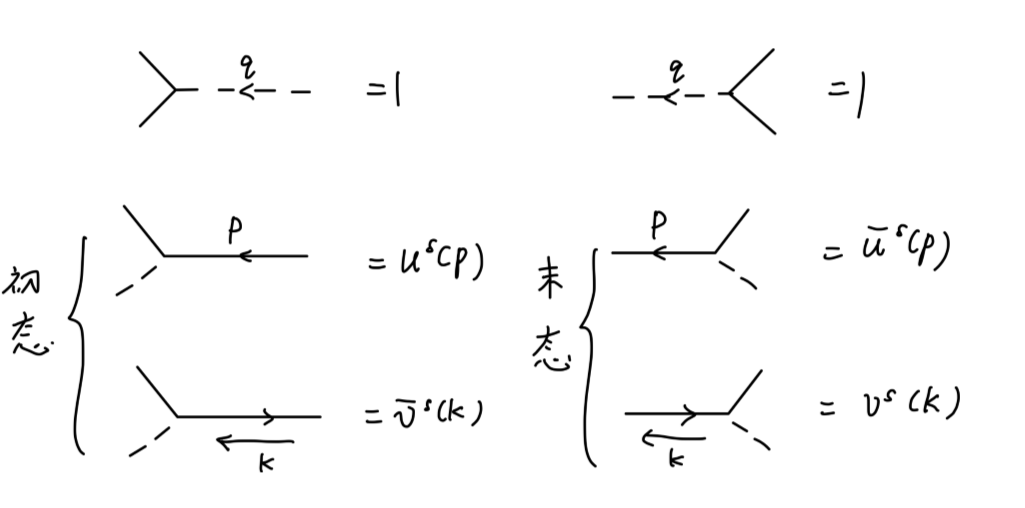
⟨p′,k′∣T{12!(−ig)∫d4xψˉIψIϕI(−ig)∫d4yψˉIψIϕI}∣p,k⟩(13)\langle \bm{p}',\bm{k}'|T\{\frac{1}{2!}(-ig)\int d^4x\bar{\psi}_I\psi_I\phi_I (-ig)\int d^4y\bar{\psi}_I\psi_I\phi_I\}|\bm{p},\bm{k}\rangle \tag{13}

之前我们已经讨论了：费米子场之间的缩并、玻色子场之间的缩并。由于在该过程中：初态/末态均为费米子，那么玻色子场不能与费米子的初态/末态发生缩并，那么费米子场能不能与费米子的初态/末态发生缩并呢？答案是肯定的。

我们需要去区分初态/末态粒子为正粒子或是反粒子。

对于正粒子有：

ψ+(x)∣p,s⟩=∫d3q(2π)312Eq∑raqrur(q)e−iq⋅x2Epaps†∣0⟩=∫d3q(2π)312Eq∑rur(q)e−iq⋅x2Ep(2π)3δrsδ(3)(p−q)∣0⟩=e−ip⋅xus(p)∣0⟩⟨p,s∣ψˉ−(x)=⟨0∣uˉs(p)eip⋅x\begin{aligned} \psi^+(x)|\bm{p},s\rangle &= \int \frac{d^3q}{(2\pi)^3} \frac{1}{\sqrt{2E_{\bm{q}}}}\sum_r a_{\bm{q}}^r u^r(\bm{q}) e^{-iq\cdot x} \sqrt{2E_{\bm{p}}} a^{s\dagger}_{\bm{p}} |0\rangle \\ & = \int \frac{d^3q}{(2\pi)^3} \frac{1}{\sqrt{2E_{\bm{q}}}}\sum_r u^r(\bm{q}) e^{-iq\cdot x} \sqrt{2E_{\bm{p}}} (2\pi)^3\delta_{rs}\delta^{(3)}(\bm{p}-\bm{q}) |0\rangle \\ & = e^{-ip\cdot x} u^{s}(p) |0\rangle \\ \langle \bm{p},s|\bar{\psi}^-(x) &= \langle 0| \bar{u}^s(p) e^{ip\cdot x}\\ \end{aligned}

对于反粒子来说：

∣k,s⟩=2Ekbks∣0⟩|\bm{k},s\rangle = \sqrt{2E_{\bm{k}}}b^s_{\bm{k}}|0\rangle

类似得到：

ψˉ+(x)∣k,s⟩=e−ik⋅xvˉs(k)∣0⟩⟨k,s∣ψ−(x)=⟨0∣vs(k)eik⋅x\begin{aligned} \bar{\psi}^+(x)|\bm{k},s\rangle &= e^{-ik\cdot x} \bar{v}^{s}(k) |0\rangle\\ \langle \bm{k},s|\psi^-(x) &= \langle 0| v^s(k) e^{ik\cdot x}\\ \end{aligned}

于是我们可以定义费米子场与费米子初态/末态间的缩并：

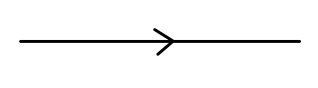
ψ∙(x)∣p,s∙⟩⏟fermion=e−ip⋅xus(p)∣0⟩⟨p,s∙∣⏟fermionψˉ∙(x)=⟨0∣uˉs(p)eip⋅xψˉ∙(x)∣k,s∙⟩⏟antifermion=e−ik⋅xvˉs(k)∣0⟩⟨k,s∙∣⏟antifermionψ∙(x)=⟨0∣vs(k)eik⋅x(14)\begin{aligned} \psi^{\bullet}(x)\underbrace{|\bm{p},s^{\bullet} \rangle}_{fermion} &= e^{-ip\cdot x} u^{s}(p) |0\rangle\\ \underbrace{\langle \bm{p},s^{\bullet}|}_{fermion} \bar{\psi}^{\bullet}(x) &= \langle 0| \bar{u}^s(p) e^{ip\cdot x}\\ \bar{\psi}^{\bullet}(x)\underbrace{|\bm{k},s^{\bullet} \rangle}_{antifermion} &= e^{-ik\cdot x} \bar{v}^{s}(k) |0\rangle\\ \underbrace{\langle \bm{k},s^{\bullet}|}_{antifermion} \psi^{\bullet}(x) &= \langle 0| v^s(k) e^{ik\cdot x}\\ \end{aligned}\tag{14}

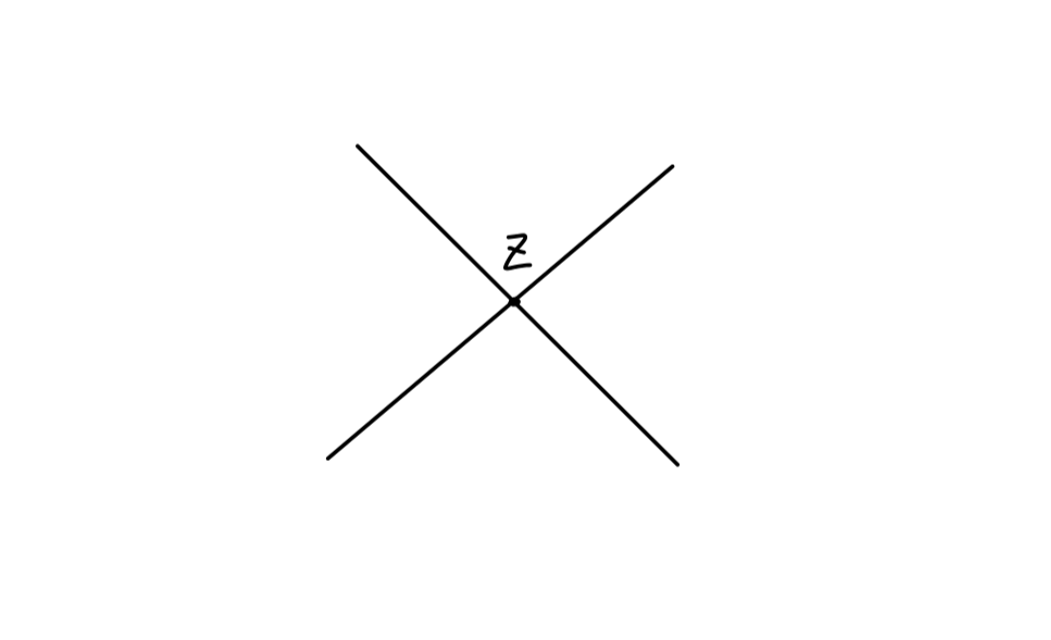
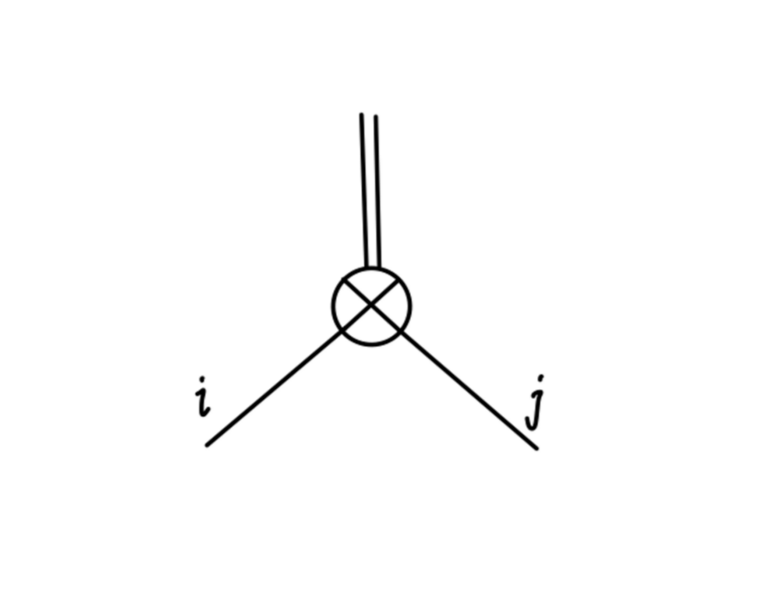
以及对应的费曼图：

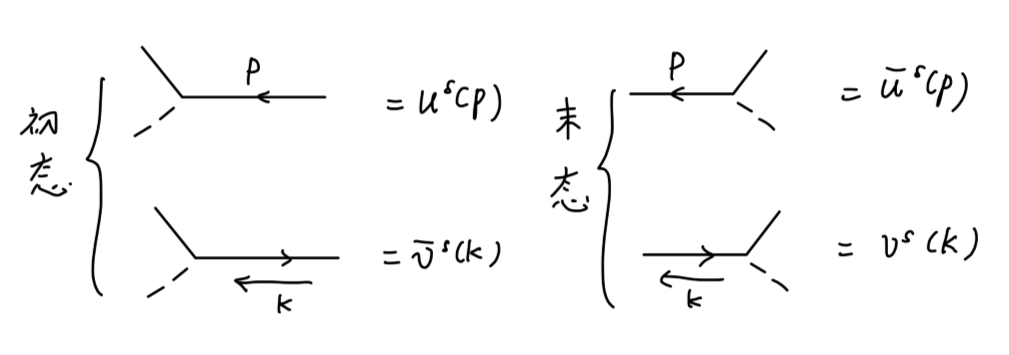
注意此时：实线代表费米子，费米子线上的箭头不再代表动量方向。我们规定：箭头沿着时间方向代表正粒子、箭头逆着时间方向代表反粒子。

Fig：费米子

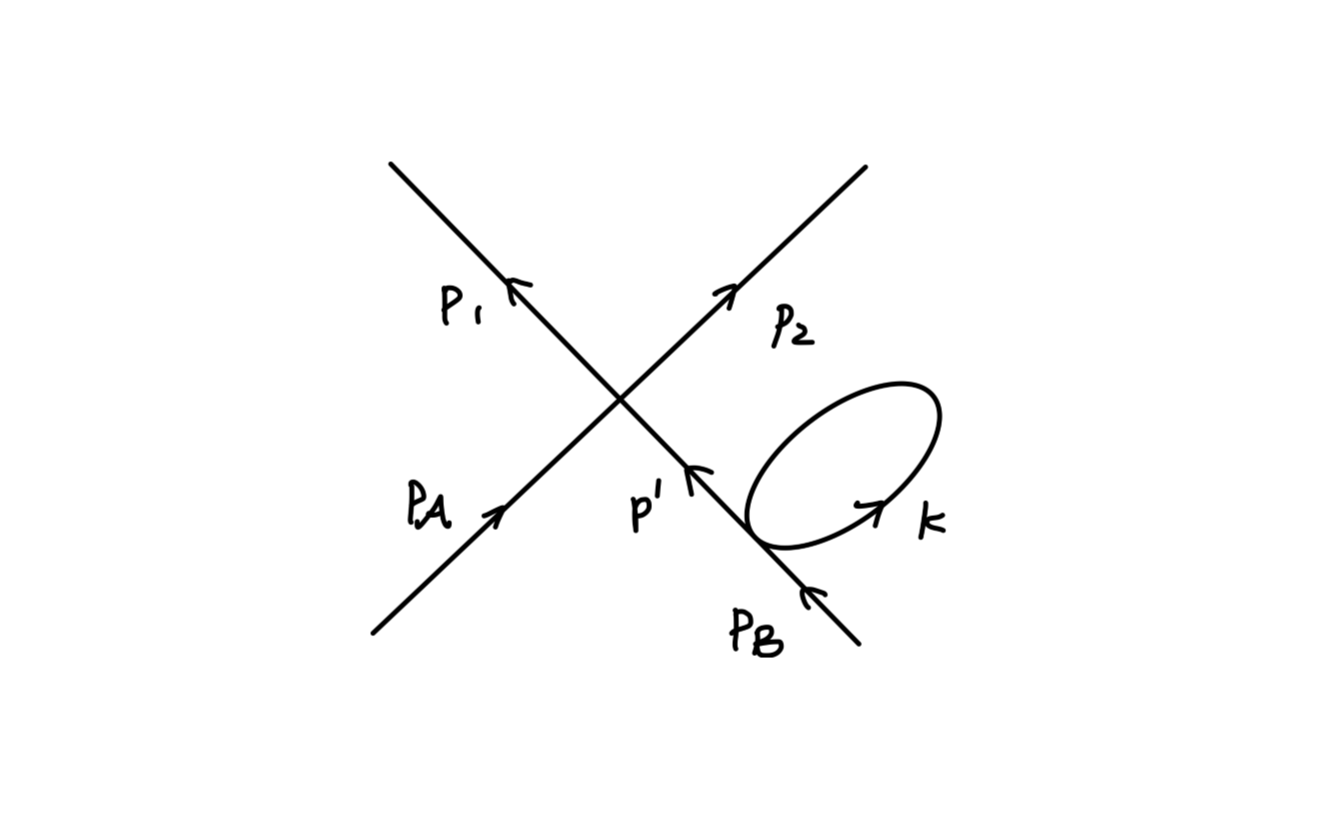
现在我们可以计算 (13)(13) 式，其为以下缩并的贡献。考虑两个顶点的对称性，存在两种等价的缩并方式

⟨p′∙,k′∙∙∣12!(−ig)∫d4xψˉI∙ψI⋆ϕI(3∙)(−ig)∫d4yψˉI∙∙ψI⋆⋆ϕI(3∙)∣p⋆,k⋆⋆⟩\langle \bm{p}'^{\bullet},\bm{k}'^{\bullet\bullet}|\frac{1}{2!}(-ig)\int d^4x\bar{\psi}_I^{\bullet}\psi_I^{\star}\phi_I^{(3\bullet)} (-ig)\int d^4y\bar{\psi}_I^{\bullet\bullet}\psi_I^{\star\star}\phi_I^{(3\bullet)}|\bm{p}^{\star},\bm{k}^{\star\star}\rangle

费曼图为：

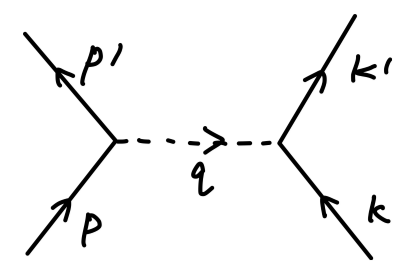
其中虚线对应 Klein-Gordon 玻色子。

其值为：

(−ig)2∫d4q(2π)4iq2−mϕ2(2π)4δ(p′−p−q)(2π)4δ(k′−k+q)uˉ(p′)u(p)uˉ(k′)u(k)(-ig)^2 \int \frac{d^4q}{(2\pi)^4}\frac{i}{q^2-m_\phi^2}(2\pi)^4\delta(p'-p-q)(2\pi)^4\delta(k'-k+q)\bar{u}(p')u(p)\bar{u}(k')u(k)

其中 mϕm_{\phi} 为 Klein-Gordon 玻色子的质量。

我们现在来推导动量空间中的费曼规则。我们采取类似的做法：对于可能出现的 Klein-Gordon 传播子：

DF(x−y)=∫d4p(2π)4ip2−mϕ2+iϵe−ip⋅(x−y)D_F(x-y) = \int \frac{d^4p}{(2\pi)^4} \frac{i}{p^2-m^2_{\phi} +i\epsilon} e^{-ip\cdot(x-y)}

或是 Dirac 传播子：

SF(x−y)=∫d4p(2π)4i(p+m)p2−m2+iϵe−ip⋅(x−y)S_F(x-y) = \int \frac{d^4p}{(2\pi)^4}\frac{i(\cancel{p}+m)}{p^2-m^2 + i\epsilon} e^{-ip\cdot(x-y)}

我们将 e−ipx,eipye^{-ipx},e^{ipy} 因子与相应的顶点关联起来。对于每个顶点，我们需要作积分，其结果为保证四动量守恒的 δ\delta 函数与耦合常数 −ig-ig 的乘积。对于每个外部线，我们也将 e−ip⋅xe^{-ip\cdot x} 与顶点关联起来，保留旋量场 u(p)u(p) 等。最后，我们要对所有未确定的动量进行积分。

另外还要说明两点：

  1. 对于 nn 阶修正中的 1/n!1/n! 因子，总可以通过对 nn 个顶点进行置换消去。

  2. Yukawa 理论对应的费曼图的对称因子 S=1S=1，因为每个顶点由 ψˉψϕ\bar{\psi}\psi\phi 组成，是不具有对称性的。

我们总结得到 Yukawa 理论动量空间的费曼规则为：

  • 传播子
    Klein-Gordon 传播子

    其值为

    iq2−mϕ2+iϵ\frac{i}{q^2-m_{\phi}^2 + i\epsilon}

    Dirac 传播子

    其值为

    i(p+m)p2−m2+iϵ\frac{i(\cancel{p}+m)}{p^2-m^2 + i\epsilon}

  • 顶点

    其值为

    −ig-ig

  • 外部线

  • 顶点保证动量守恒。

  • 对未确定的动量积分：

∫d4p(2π)4\int \frac{d^4p}{(2\pi)^4}

  • 确定图的符号。

现在我们来讨论以下最后一点：图的符号。对于多个粒子构成初态与末态来说，我们习惯取：

∣p,k⟩∼ap†ak†∣0⟩,⟨p′,k′∣∼⟨0∣ak′ap′|\bm{p},\bm{k}\rangle \sim a_{\bm{p}}^\dagger a_{\bm{k}}^\dagger |0\rangle,\quad \langle \bm{p}',\bm{k}'| \sim \langle 0|a_{\bm{k}'} a_{\bm{p}'}

这里的阶梯算子的顺序是关键的，如此有：∣p,k⟩†=⟨p,k∣|\bm{p},\bm{k}\rangle^\dagger = \langle \bm{p},\bm{k}|

那么考虑两个全同费米子的散射的领头阶：

⟨p′,k′∣(ψˉψ)x(ψˉψ)y∣p,k⟩\langle \bm{p}',\bm{k}'| (\bar{\psi}\psi)_x(\bar{\psi}\psi)_y |\bm{p},\bm{k}\rangle

存在两种不等价的缩并方式：

这里的玻色子场，耦合常数，积分等元素暂时略去了。我们重点关注费米子场的缩并。

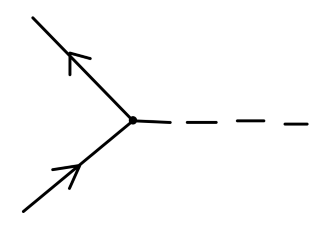
⟨p′∙,k′∙∙∣(ψˉ∙∙ψ⋆⋆)x(ψˉ∙ψ⋆)y∣p⋆,k⋆⋆⟩⟨p′∙,k′∙∙∣(ψˉ∙ψ⋆⋆)x(ψˉ∙∙ψ⋆)y∣p⋆,k⋆⋆⟩\begin{aligned} \langle \bm{p}'^{\bullet},\bm{k}'^{\bullet\bullet}| (\bar{\psi}^{\bullet\bullet}\psi^{\star\star})_x(\bar{\psi}^{\bullet}\psi^{\star})_y |\bm{p}^{\star},\bm{k}^{\star\star}\rangle\\ \langle \bm{p}'^{\bullet},\bm{k}'^{\bullet\bullet}| (\bar{\psi}^{\bullet}\psi^{\star\star})_x(\bar{\psi}^{\bullet\bullet}\psi^{\star})_y |\bm{p}^{\star},\bm{k}^{\star\star}\rangle\\ \end{aligned}

它们的值相差一个负号。对应的费曼图分别为：

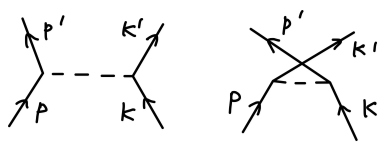
它们对全同费米子的散射都有贡献。

iM=(−ig2)[uˉ(p′)u(p)1(p′−p)2−mϕ2uˉ(k′)u(k)−uˉ(p′)u(k)1(p−k′)2−mϕ2uˉ(k′)u(p)]\begin{aligned} i\mathcal{M} = &(-ig^2)[\bar{u}(p')u(p)\frac{1}{(p'-p)^2-m_{\phi}^2}\bar{u}(k')u(k)\\ &-\bar{u}(p')u(k)\frac{1}{(p-k')^2-m_{\phi}^2}\bar{u}(k')u(p)] \end{aligned}

对于一些较为复杂的情形，我们常常将 ψˉψ\bar{\psi}\psi 组合在一起考虑以简化计算。例如：

⋯(ψˉψ∙)x(ψˉ∙∙ψ⋆)y(ψˉ∙ψ∙∙)z(ψˉ⋆ψ)w⋯=⋯(+1)(ψˉψ∙)x(ψˉ∙ψ∙∙)z(ψˉ∙∙ψ⋆)y(ψˉ⋆ψ)w⋯=⋯SF(x−z)SF(z−y)SF(y−w)⋯\begin{aligned} &\cdots (\bar{\psi}\psi^{\bullet})_x (\bar{\psi}^{\bullet\bullet}\psi^{\star})_y (\bar{\psi}^{\bullet}\psi^{\bullet\bullet})_z (\bar{\psi}^{\star}\psi)_w \cdots\\ =& \cdots (+1) (\bar{\psi}\psi^{\bullet})_x (\bar{\psi}^{\bullet}\psi^{\bullet\bullet})_z (\bar{\psi}^{\bullet\bullet}\psi^{\star})_y (\bar{\psi}^{\star}\psi)_w \cdots\\ =& \cdots S_F(x-z)S_F(z-y)S_F(y-w)\cdots \end{aligned}

对于如下圈图：

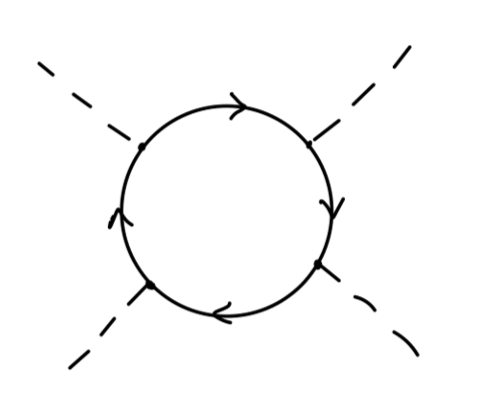
其值为：

ψˉ⋆⋆ψ∙ ψˉ∙ψ∙∙ ψˉ∙∙ψ⋆ ψˉ⋆ψ⋆⋆=−tr[ψ⋆⋆ψˉ⋆⋆ψ∙ ψˉ∙ψ∙∙ ψˉ∙∙ψ⋆ ψˉ⋆]=−tr[SFSFSFSF](15)\begin{aligned} &\bar{\psi}^{\star\star}\psi^{\bullet}\ \bar{\psi}^{\bullet}\psi^{\bullet\bullet}\ \bar{\psi}^{\bullet\bullet}\psi^{\star}\ \bar{\psi}^{\star}\psi^{\star\star}\\ =& -\mathrm{tr} [\psi^{\star\star}\bar{\psi}^{\star\star}\psi^{\bullet}\ \bar{\psi}^{\bullet}\psi^{\bullet\bullet}\ \bar{\psi}^{\bullet\bullet}\psi^{\star}\ \bar{\psi}^{\star}]\\ =&- \mathrm{tr} [ S_FS_FS_FS_F]\\ \end{aligned}\tag{15}

Yukawa 势

我们现在在非相对论极限下对上述散射的计算做一些估计。我们此时只保留含有三维动量的最低阶项。有：

p=(m,p)k=(m,k)p′=(m,p′)k′=(m,k′)\begin{aligned} &p = (m,\bm{p})\quad &k = (m,\bm{k})\\ &p' = (m,\bm{p}')\quad &k' = (m,\bm{k}')\\ \end{aligned}

以及：

(p′−p)2=−∣p′−p∣2+O(p4)us(p)=m(ξsξs)\begin{aligned} &(p'-p)^2 = -|\bm{p}'-\bm{p}|^2 + \mathcal{O}(\bm{p}^4)\\ &u^s(p) = \sqrt{m}\begin{pmatrix} \xi^s\\ \xi^s\\ \end{pmatrix}\\ \end{aligned}

旋量积具有如下关系：

uˉs′(p′)us(p)=2mξs′†ξs=2mδss′uˉr′(p′)ur(p)=2mξr′†ξr=2mδrr′\begin{aligned} \bar{u}^{s'}(p')u^s(p) = 2m\xi^{s'\dagger}\xi^s = 2m\delta^{ss'}\\ \bar{u}^{r'}(p')u^r(p) = 2m\xi^{r'\dagger}\xi^r = 2m\delta^{rr'}\\ \end{aligned}

那么对应的跃迁矩阵元成为：

iM=(−ig2)uˉ(p′)u(p)1(p′−p)2−mϕ2uˉ(k′)u(k)=ig2∣p′−p∣2+mϕ22mδss′2mδrr′(16)\begin{aligned} i\mathcal{M} = &(-ig^2)\bar{u}(p')u(p)\frac{1}{(p'-p)^2-m_{\phi}^2}\bar{u}(k')u(k)\\ = &\frac{ig^2}{|\bm{p}'-\bm{p}|^2+m_{\phi}^2}2m\delta^{ss'}2m\delta^{rr'}\\ \end{aligned}\tag{16}

在非相对论量子力学中，我们利用 Born 近似可以写出跃迁矩阵元：

⟨p′∣iT∣p⟩=−iV~(q)(2π)δ(Ep′−Ep)q=p′−p(17)\langle p'|iT|p\rangle = -i\tilde{V}(\bm{q})(2\pi)\delta(E_{\bm{p}'}-E_{\bm{p}})\quad \bm{q} = \bm{p}'-\bm{p} \tag{17}

比较 (16)(17)(16)(17) 式，我们得到 Yukawa 相互作用的势函数形式为：

V~(q)=−g2∣q∣2+mϕ2(18)\tilde{V}(\bm{q}) = \frac{-g^2}{|\bm{q}|^2 + m_{\phi}^2} \tag{18}

变换到坐标空间，得到：

V(r)=−g24π1re−mϕr(19)V(r) = -\frac{g^2}{4\pi}\frac{1}{r}e^{-m_{\phi}r} \tag{19}

这就是 Yukawa 势 Yukawa potential。

QED

下面我们从 Yuakawa 理论过渡到 QED(Quantum Electrodynamics)。此时只需要将 Yukawa 相互作用项中的标量场 ϕ\phi 替换为矢量场 AμA_{\mu} 即可：

Hint=∫d3xeψˉγμψAμ(20)H_{int} = \int d^3x e\bar{\psi}\gamma^{\mu}\psi A_{\mu} \tag{20}

我们考虑矢势的洛伦兹规范：

∂2Aμ=0(21)\partial^2 A_{\mu} = 0 \tag{21}

那么实际上 AμA_{\mu} 满足无质量 Klein-Gordon 方程，类似的，可以将电磁场量子化：

Aμ(x)=∫d3p(2π)312Ep∑r=03(aprϵμr(p)e−ip⋅x+apr†ϵμr∗(p)eip⋅x)(22)A_{\mu}(x) = \int \frac{d^3p}{(2\pi)^3}\frac{1}{\sqrt{2E_{\bm{p}}}}\sum_{r=0}^{3}(a_{\bm{p}}^r\epsilon_{\mu}^r(p)e^{-ip\cdot x}+a_{\bm{p}}^{r\dagger}\epsilon_{\mu}^{r*}(p)e^{ip\cdot x})\tag{22}

QED 的费曼规则我们不作证明，但它们的形式是容易理解的。我们现在需要补充有关电磁场的矢量玻色子——光子的有关费曼规则：

  • 光子传播子

    值为：

    −gμνq2+iϵ\frac{-g_{\mu\nu}}{q^2+i\epsilon}

  • 顶点

    值为：

    −ieγμ-ie\gamma^{\mu}

  • 外部光子线

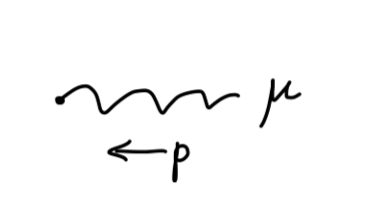
    值为：

    Aμ∙∣p∙⟩=ϵμ(p)A^{\bullet}_{\mu}|\bm{p}^{\bullet}\rangle = \epsilon_{\mu}(p)

    值为：

    ⟨p∙∣Aμ∙=ϵμ∗(p)\langle \bm{p}^{\bullet}|A_{\mu}^{\bullet} = \epsilon^*_{\mu}(p)

其中 ϵμ(p)\epsilon_{\mu}(p) 为光子的极化矢量。

类似 Yukawa 势，可以推导得到非相对论极限下 库伦势 Coulomb Potential的表达式：

V(r)=e24πrV(r) = \frac{e^2}{4\pi r}